5.4 Boundary conditions for 2nd order PDEs

$$ \begin{align} \frac{\partial^2u}{\partial x^2}+\frac{\partial^2u}{\partial y^2} & =0: \text{Elliptic . No real characteristics.} \tag{5.38}\\ \frac{\partial^2u}{\partial x^2}-\frac{\partial^2u}{\partial y^2} & =0: \text{Hyperbolic. Two rel characteristics.} \tag{5.39}\\ \frac{\partial u}{\partial y} & =\frac{\partial^2 u}{\partial x^2}: \text {Parabolic. One real characteristic.} \tag{5.40} \end{align} $$

The model equations shown above differ by the number of real characteristics. This directly affects the boundary conditions that are possible in the three cases, as physical information propagates along characteristics.

5.4.1 Hyperbolic equations

As example we use the wave equation: $$ \begin{equation} \frac{\partial^2u}{\partial t^2}=\alpha_0^2\frac{\partial^2u}{\partial x^2} \tag{5.41} \end{equation} $$

(5.41) has the characteristic $ \dfrac{dx}{dt}=\pm a_0 $, where $ a_0 $ is the wave propagation speed. We denote $ \dfrac{dx}{dt}=+a_0 $ with $ C^+ $ and $ \dfrac{dx}{dt}=-a_0 $ with $ C^- $. The area of influence for (5.41) is shown in Figure 70.

Figure 70: Regions of dependence and influence with respect to point $ P $ for the wave equation(5.41).

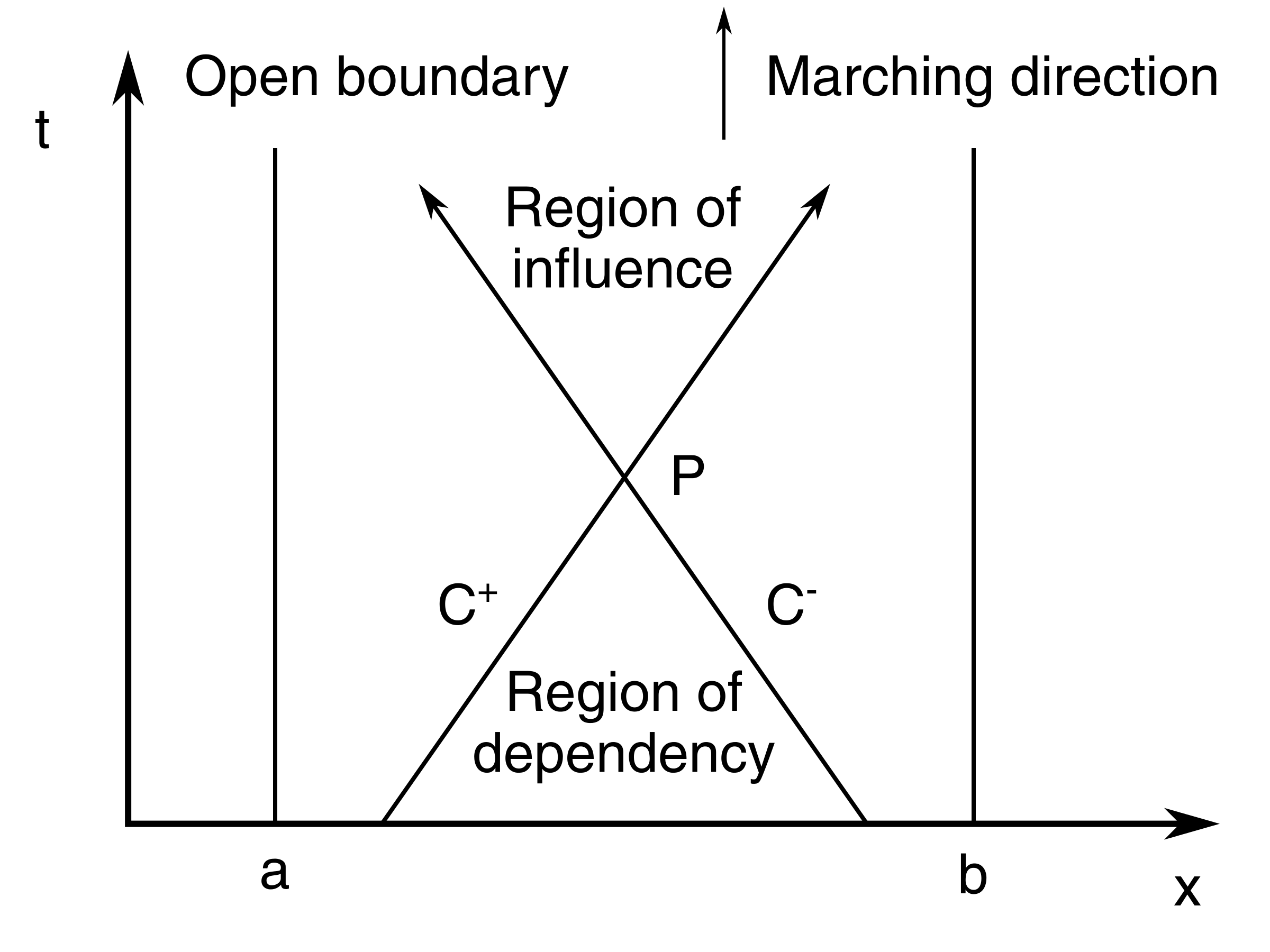
The solution at point $ P $ depends only on the solution in the region of dependence, while the value at $ P $ only affects the solution within the region of influence. Boundary values for domain borders can not be prescribed independently of initial conditions at $ t=0 $. In order to solve this equation as an initial value problem, (5.41) must also have an initial condition for $ \dfrac{\partial u}{\partial t} $ at $ t=0 $.

5.4.2 Elliptic equations

Model equation: $$ \begin{equation} \frac{\partial^2u}{\partial x^2}+\frac{\partial^2u}{\partial y^2}=0 \tag{5.42} \end{equation} $$ The solution domain is shown in Figure 71.

Figure 71: Regions of dependence and influence for the model elliptic equation (5.42).

We have no real characteristics. The entire domain $ \Omega $, including its boundary $ C $, coincide with the regions of dependence and influence for $ P $: Any change of a value in $ \Omega $ or $ C $ will affect the solution in $ P $. (5.42) is a purely boundary value problem and the following boundary conditions are admissible:

$ u $ is prescribed at C: Dirichlet boundary condition.
$ \dfrac{\partial u}{\partial n} $ is prescribed at $ C $: Neumann boundary condition.
a weighted combination of $ u $ and $ \dfrac{\partial u}{\partial n} $ are prescribed at $ C $: Robin boundary condition.
a combination of the above conditions in different portions of $ C $.

5.4.3 Parabolic equations

Model equation: $$ \begin{equation} \frac{\partial u}{\partial t}=\frac{\partial^2u}{\partial x^2} \tag{5.43} \end{equation} $$ The solution domain for (5.43) is shown in Figure 72.

Figure 72: Regions of dependence and influence for the model parabolic equation (5.43).

From the classification equations (5.34) we find that:

$ dt=0\Rightarrow t=\mathrm{constant} $ is the characteristic curve in this case. Hence: $ a_0=\frac{dx}{dt}=\infty $, which means that the propagation velocity along characteristic $ t=\mathrm{constant} $ is infinitely large. The solution at $ P $ depends on the value in all points in the physical space for past time $ t $, including the present. (5.43) behaves like an elliptical equation for each value of $ t=\mathrm{constant} $.