Example A.4: Linear Advection with Different Limiters
The linear advection problem with periodic boundary conditions
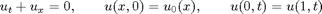
is well suited for studying error mechanisms in numerical schemes for hyperbolic conservation laws. Here, we will use initial data consisting of a combination of a smooth, squared cosine wave and double step function to study the compressive and dissipative nature of four different limiters for a second-order nonoscillatory central-difference scheme.
If we choose the CFL number exactly equal to the stability limit of 0.5, the central scheme will produce solutions with excellent accuracy regardless of our choice of limiter. In practical computations, however, one cannot expect to simulate a linear wave with a CFL number equal the stability limit of the scheme. Hence, we choose a somewhat lower number to exhibit the typical behavior of the various limiters.
T = 20; CFL = 0.475; N = 100; h=1/N; x=h*(1:N); u0 = (abs(x-.25)<=0.15).*(cos(pi*(10/3)*(x-0.25))).^2+... 1.0*(abs(x-0.75)<0.15); xx = linspace(0,1,1001); uf = (abs(xx-.25)<=0.15).*(cos(pi*(10/3)*(xx-0.25))).^2+... 1.0*(abs(xx-0.75)<0.15);
We consider four limiters (MinMod, vanLeer, MacCormack, and Superbee) and study the solution after 20 'passes' over the unit interval.
limiter = {'minmod', 'vanleer', 'mc', 'superbee'};
name = {'MinMod', 'vanLeer', 'MacCormack', 'Superbee'};
for i=1:4
u=central('linearfunc',u0,T,h,1,'periodic',CFL,limiter{i});
subplot(2,2,i);
plot(xx,uf,'-',x,u,'o','MarkerSize',4); axis([0 1 -0.2 1.3]);
title(name{i});
end

The MinMod limiter is clearly dissipative, clipping the top of the smooth wave and smoothing the discontinuities of the double step, and in this way behaves somewhat like classical first-order scheme. The superbee limiter, on the other hand, picks steeper slopes and can thus resolve the discontinuities using very few cells but has also a tendency of overcompressing smooth linear waves, as observed for the smooth cosine profile. The other two limiters are somewhere in between.