tt-geometry in Trondheim
- Trondheim, Norway
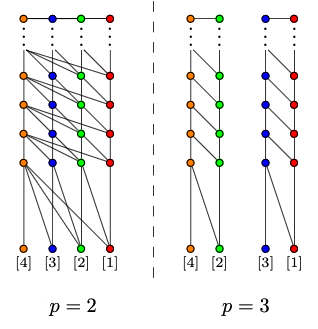
This is the project page for the project `tt-geometry in Trondheim', supported by the Trond Mohn Foundation. For background reading on tensor-triangulated geometry, a good introduction can be found in the survey papers of Balmer:
A recent online lecture given by Drew Heard can be found here.
The image on the left is part of a recent computation, joint with Arone, Barthel, and Sanders.
Members

Drew Heard
Drew is a førsteamanuensis (associate professor) at Trondheim University supported by a grant from the Trond Mohn Foundation. Previously he has been a postdoc at Regensburg University, a postdoc at Haifa University, a guest researcher at Universität Hamburg, and a guest at the Max Planck Institute for Mathematics in Bonn, Germany. His research is mainly focused on tensor-triangulated geometry and chromatic homtopy theory.

Clover May
Clover is a postdoc at Trondheim University supported by a grant from the Trond Mohn Foundation. Previously she was a postdoc at UCLA. Her work is in equivariant homotopy theory with a focus on \(RO(G)\)-graded Bredon cohomology, structured ring spectra, and tensor triangular geometry.

Torgeir Aambø
Torgeir is a PhD student at Trondheim University supported by a grant from the Trond Mohn Foundation. He is working on a project relating to algebraic models of triangulated categories. He also maintains an active blog on mathematics.

Marius Verner Bach Nielsen
Marius is a PhD student at Trondheim University supported in part by a grant from the Trond Mohn Foundation. He is currently working on the the relationship between Goodwillie calculus and spectral Mackey functors, and has previously thought about the \(Q\)-shaped derived category.
Publications
Descent in tensor triangular geometry - Tobias Barthel, Natalia Castellana, Drew Heard Niko Naumann, Luca Pol, and Beren Sanders. To appear in The Abel Symposium 2022
We investigate to what extent we can descend the classification of localizing, smashing and thick ideals in a presentably symmetric monoidal stable \(\infty\)-category \( \mathcal{C} \) along a descendable commutative algebra \(A\). We establish equalizer diagrams relating the lattices of localizing and smashing ideals of \( \mathcal{C} \) to those of \( \newcommand{\Mod}{\mathop{\rm Mod}\nolimits} \Mod_{A}(\mathcal{C})\) and \(\Mod_{A \otimes A}(\mathcal{C})\). If \(A\) is compact, we obtain a similar equalizer for the lattices of thick ideals which, via Stone duality, yields a coequalizer diagram of Balmer spectra in the category of spectral spaces. We then give conditions under which the telescope conjecture and stratification descend from \(\Mod_{A}(\mathcal{C})\) to \(\mathcal{C}\). The utility of these results is demonstrated in the case of faithful Galois extensions in tensor triangular geometry.
Invertible objects in Franke's comodule categories. - Drew Heard. To appear in Mathematica Scandinavica
We study the Picard group of Franke's category of quasi-periodic \(E_0E\)-comodules for \(E\) a 2-periodic Landweber exact cohomology theory of height \(n\) such as Morava \(E\)-theory, showing that for \(2p−2>n^2+n\), this group is infinite cyclic, generated by the suspension of the unit. This is analogous to, but independent of, the corresponding calculations by Hovey and Sadofsky in the \(E\)-local stable homotopy category. We also give a computation of the Picard group of \(I_n\)-complete quasi-periodic \(E_0E\)-comodules when \(E\) is Morava \(E\)-theory, as studied by Barthel--Schlank--Stapleton for \(2p−2 \ge n^2\) and \(p−1 \nmid n\), and compare this to the Picard group of the \(K(n)\)-local stable homotopy category, showing that they agree up to extension.
Equivariant \( \underline{\mathbb{Z}/\ell} \)-modules for the cyclic group \( C_2 \) - Daniel Dugger, Christy Hazel, and Clover May. Journal of Pure and Applied Algebra (arXiv link)
For the cyclic group \(C_2\) we give a complete description of the derived category of perfect complexes of modules over the constant Mackey ring \( \mathbb{Z}/\ell \) for \( \ell \) a prime. This is fairly simple for \( \ell \) odd, but for \( \ell = 2 \) depends on a new splitting theorem. As corollaries of the splitting theorem we compute the associated Picard group and the Balmer spectrum for compact objects in the derived category, and we obtain a complete classification of finite modules over the \( C_2 \)-equivariant Eilenberg--MacLane spectrum \( H\underline{\mathbb{Z}/2}\). We also use the splitting theorem to give new and illuminating proofs of some facts about \(RO(C2)\)-graded Bredon cohomology, namely Kronholm's freeness theorem and the structure theorem of C. May.
Stratification in tensor triangular geometry with applications to spectral Mackey functors - Tobias Barthel, Drew Heard, and Beren Sanders. Cambridge Journal of Mathematics (arXiv link)
We systematically develop a theory of stratification in the context of tensor triangular geometry and apply it to classify the localizing tensor-ideals of certain categories of spectral G-Mackey functors for all finite groups G. Our theory of stratification is based on the approach of Stevenson which uses the Balmer--Favi notion of big support for tensor-triangulated categories whose Balmer spectrum is weakly noetherian. We clarify the role of the local-to-global principle and establish that the Balmer--Favi notion of support provides the universal approach to weakly noetherian stratification. This provides a uniform new perspective on existing classifications in the literature and clarifies the relation with the theory of Benson--Iyengar--Krause. Our systematic development of this approach to stratification, involving a reduction to local categories and the ability to pass through finite \'{e}tale extensions, may be of independent interest. Moreover, we strengthen the relationship between stratification and the telescope conjecture. The starting point for our equivariant applications is the recent computation by Patchkoria--Sanders--Wimmer of the Balmer spectrum of the category of derived Mackey functors, which was found to capture precisely the height \(0\) and height \(\infty\) chromatic layers of the spectrum of the equivariant stable homotopy category. We similarly study the Balmer spectrum of the category of \(E(n)\)-local spectral Mackey functors noting that it bijects onto the height \(\le n\) chromatic layers of the spectrum of the equivariant stable homotopy category; conjecturally the topologies coincide. Despite our incomplete knowledge of the topology of the Balmer spectrum, we are able to completely classify the localizing tensor-ideals of these categories of spectral Mackey functors.
Stratification and the comparison between homological and tensor triangular support - Tobias Barthel, Drew Heard, and Beren Sanders. The Quarterly Journal of Mathematics (arXiv link)
We compare the homological support and tensor triangular support for `big' objects in a rigidly-compactly generated tensor triangulated category. We prove that the comparison map from the homological spectrum to the tensor triangular spectrum is a bijection and that the two notions of support coincide whenever the category is stratified, extending work of Balmer. Moreover, we clarify the relations between salient properties of support functions and exhibit counter-examples highlighting the differences between homological and tensor triangular support.
The \(\mathop{Sp}_{k,n}\)-local stable homotopy category - Drew Heard Algebraic & Geometric Topology (arXiv link)
Following a suggestion of Hovey and Strickland, we study the category of \(K(k) \vee K(k+1) \vee \cdots \vee K(n)\)-local spectra. When \(k = 0\), this is equivalent to the category of \(E(n)\)-local spectra, while for \(k = n\), this is the category of \(K(n)\)-local spectra, both of which have been studied in detail by Hovey and Strickland. Based on their ideas, we classify the localizing and colocalizing subcategories, and give characterizations of compact and dualizable objects. We construct an Adams type spectral sequence and show that when \(p \gg n\) it collapses with a horizontal vanishing line above filtration degree \(n^2+n-k\) at the \(E_2\)-page for the sphere spectrum. We then study the Picard group of \(K(k) \vee K(k+1) \vee \cdots \vee K(n)\)-local spectra, showing that this group is algebraic, in a suitable sense, when \(p \gg n\). We also consider a version of Gross--Hopkins duality in this category. A key concept throughout is the use of descent.
On conjectures of Hovey–Strickland and Chai - Tobias Barthel, Drew Heard, and Niko Naumann. Selecta Mathematica (arXiv link)
We prove the height two case of a conjecture of Hovey and Strickland that provides a \(K(n)\)-local analogue of the Hopkins–Smith thick subcategory theorem. Our approach first reduces the general conjecture to a problem in arithmetic geometry posed by Chai. We then use the Gross &ndash Hopkins period map to verify Chai's Hope at height two and all primes. Along the way, we show that the graded commutative ring of completed cooperations for Morava \(E\)-theory is coherent, and that every finitely generated Morava module can be realized by a \(K(n)\)-local spectrum as long as \(2p-2>n^2+n\). Finally, we deduce consequences of our results for descent of Balmer spectra.
Rational local systems and connected finite loop spaces - Drew Heard. Glasgow Mathematical Journal (arXiv link)
Greenlees has conjectured that the rational stable equivariant homotopy category of a compact Lie group always has an algebraic model. Based on this idea, we show that the category of rational local systems on a connected finite loop space always has a simple algebraic model. When the loop space arises from a connected compact Lie group, this recovers a special case of a result of Pol and Williamson about rational cofree \(G\)-spectra. More generally, we show that if \(K\) is a closed subgroup of a compact Lie group \(G\) such that the Weyl group \(W_GK\) is connected, then a certain category of rational \(G\)-spectra `at \(K\)' has an algebraic model. For example, when \(K\) is the trivial group, this is just the category of rational cofree \(G\)-spectra, and this recovers the aforementioned result. Throughout, we pay careful attention to the role of torsion and complete categories.
Preprints
Algebraicity in monochromatic homotopy theory - Torgeir Aambø.
Using Patchkoria--Pstrągowski's version of Franke's algebraicity theorem, we prove that the category of \(K_p(n)\)-local spectra is exotically equivalent to the category of derived \(I_n\)-complete periodic comodules over the Adams Hopf algebroid \((E_*,E_*E)\) for large primes. This gives a finite prime result analogous to the asymptotic algebraicity for \(Sp_{K_p(n)}\) of Barthel--Schlank--Stapleton.
The spectrum of excisive functors - Greg Arone, Tobias Barthel, Drew Heard and and Beren Sanders.
We prove a thick subcategory theorem for the category of \(d\)-excisive functors from finite spectra to spectra. This generalizes the Hopkins-Smith thick subcategory theorem (the \(d=1\) case) and the \(C_2\)-equivariant thick subcategory theorem (the \(d=2\) case). We obtain our classification theorem by completely computing the Balmer spectrum of compact \(d\)-excisive functors. A key ingredient is a non-abelian blueshift theorem for the generalized Tate construction associated to the family of non-transitive subgroups of products of symmetric groups. Also important are the techniques of tensor triangular geometry and striking analogies between functor calculus and equivariant homotopy theory. In particular, we introduce a functor calculus analogue of the Burnside ring and describe its Zariski spectrum à la Dress. The analogy with equivariant homotopy theory is strengthened further through two applications: We explain the effect of changing coefficients from spectra to \(H\mathbb{ℤ}\)-modules and we establish a functor calculus analogue of transchromatic Smith-Floyd theory as developed by Kuhn-Lloyd. Our work offers a new perspective on functor calculus which builds upon the previous approaches of Arone-Ching and Glasman.
On surjectivity in tensor triangular geometry - Tobias Barthel, Natalia Castellana, Drew Heard and Beren Sanders.
We prove that a jointly conservative family of geometric functors between rigidly-compactly generated tensor triangulated categories induces a surjective map on spectra. From this we deduce a fiberwise criterion for Balmer's comparison map to be a homeomorphism. This gives short alternative proofs of the Hopkins--Neeman theorem and Lau's theorem for the trivial action.
Cosupport in tensor triangular geometry - Tobias Barthel, Natalia Castellana, Drew Heard and Beren Sanders.
We develop a theory of cosupport and costratification in tensor triangular geometry. We study the geometric relationship between support and cosupport, provide a conceptual foundation for cosupport as categorically dual to support, and discover surprising relations between the theory of costratification and the theory of stratification. We prove that many categories in algebra, topology and geometry are costratified by developing and applying descent techniques. An overarching theme is that cosupport is relevant for diverse questions in tensor triangular geometry and that a full understanding of a category requires knowledge of both its support and its cosupport.
Quillen stratification in equivariant homotopy theory - Tobias Barthel, Natalia Castellana, Drew Heard , Niko Naumann, and Luca Pol.
We prove a version of Quillen's stratification theorem in equivariant homotopy theory for a finite group \(G\), generalizing the classical theorem in two directions. Firstly, we work with arbitrary commutative equivariant ring spectra as coefficients, and secondly, we categorify it to a result about equivariant modules. Our general stratification theorem is formulated in the language of equivariant tensor-triangular geometry, which we show to be tightly controlled by the non-equivariant tensor-triangular geometry of the geometric fixed points. We then apply our methods to the case of Borel-equivariant Lubin--Tate \(E\)-theory \(\underline{E}_n\), for any finite height \( n\) and any finite group \(G\), where we obtain a sharper theorem in the form of cohomological stratification. In particular, this provides a computation of the Balmer spectrum as well as a cohomological parametrization of all localizing \(\otimes\)-ideals of the category of equivariant modules over \(\underline{E}_n\), thereby establishing a finite height analogue of the work of Benson, Iyengar, and Krause in modular representation theory.